An Introduction to…Intervals

Understanding the basics of music theory can go a long way in helping you read music, create music, and communicate with other musicians. And Sheet Music Direct is here to help! Take a look at our introductions to other key music theory topics, including pitch, chords, and rhythm.
Intervals
The payoff of learning intervals is a much better understanding of chords and melodies.
An interval (the distance between two pitches) may be measured from the lower note to the higher note (ascending) or from the higher note to the lower note (descending). Since the distance is the same either way, the name of the interval is the same whether it is ascending or descending.
Consecutive notes create a melodic interval. Simultaneous notes create a harmonic interval. Both are measured the same way. The interval name has two parts: quantity and quality, which must be used together to determine its exact size.
Interval Quantity
The quantity of an interval is expressed with a number. To find the quantity of an interval we count the lines and spaces from one pitch to the next in either direction. We can also use the letter names, assigning one number to each letter.
With the bottom note as 1, we count up to the higher note. Here is an ascending 5th.
We can also use the alphabet to find the quantity.
If the higher note is first, we have a descending 5th.
For speed, remember that in odd-numbered intervals (1, 3, 5, 7) both notes are on a line or on a space. In even-numbered intervals (2, 4, 6, 8) one note is on a line while the other is on a space. Notice there is no "zero" interval; notes of the same pitch are 1 (unison or prime).
Interval Quality
Quality gives the exact size of an interval and is represented by a name: major, minor, perfect, diminished, or augmented. Quality is determined by comparison with the degrees of the major scale. The intervals based on the 2nd, 3rd, 6th, and 7th degrees of the major scale are major in quality:
The intervals based on the 1st, 4th, 5th, and 8th degrees of the major scale are called perfect in quality:
To create a major or perfect interval we treat the bottom note as if it were the root of a major scale. Knowing the major scale formula (1 2 3^4 5 6 7^8) and the natural half steps (B–C, E–F) is essential here.
To create an ascending major 3rd starting from C, we count up to the 3rd degree of the C major scale.
Let's do it again with a larger example. Here we find an ascending major 6th from B. Call B the root, then count up, following the major-scale formula.
Descending Intervals
To create a descending interval, we can count lines and spaces to roughly find the quantity. Then to get the correct accidental for the lower note, thus assuring the correct quality, we again use the major-scale formula, but this time counting backwards (8^7 6 5 4^3 2 1).
Let's create a descending major 3rd starting from C. Counting three lines and spaces shows the lower note will be an A of some kind. Now we count major-scale steps. From 3 to 2 is a whole step. If C is 3, then 2 must be B-flat. Why? Because C–B is a natural half step.
From 2 to 1 of the major scale is also a whole step, so we find that 1 is A-flat. (B-flat to A would only be a half step.)
Here's a larger example, a descending major 6th from B-flat . Counting lines and spaces we find a D of some kind, but this is only the quantity. By following the major-scale formula, making sure there are whole steps between all degrees, except for a half step from 4 down to 3, we find that 1 is Db.
Minor, Diminished, and Augmented Intervals
Diminution (the act of diminishing) is the reduction in quality of an interval. (Remember that quality is the exact interval size.) The opposite, augmentation, is the increase in the quality of an interval. With diminution or augmentation, we change the accidentals on the notes. The basic quantity (numeric value and letter names) of the interval stays the same.
When a major interval (2, 3, 6, 7) is reduced by a half step, it becomes minor. This smaller interval could be created by lowering the top note or by raising the bottom note.
If this interval undergoes diminution by another half step, it becomes diminished.
When a perfect interval (1, 4, 5, 8) undergoes diminution by a half step, it immediately becomes diminished in quality. There is no minor level for intervals 1, 4, 5, or 8.
Both major and perfect intervals become augmented in quality when augmented by a half step. A minor interval becomes major, then augmented in quality by going up two half steps.
A diminished interval, increased by a half step, becomes either minor or perfect, depending on which quantity of interval it is: major (2, 3, 6, 7) or perfect (1, 4, 5, 8).
Larger manipulations, resulting in “doubly-augmented” or “doubly-diminished” intervals are also possible but are rarely needed.
To construct or identify a minor, diminished, or augmented interval on the staff, we first build the major or perfect interval upon which it is based. Then we make the necessary increase or reduction.
To spell an ascending augmented 5th from G, we start with a perfect 5th from the G major scale. Augment by one half step. G to D-sharp is an augmented 5th.
To spell an ascending minor 7th from C-sharp, we find the 7th of the C-sharp major scale, then diminish (reduce) by one half step. B is the minor 7th of C-sharp.
Let's stay with C-sharp and, this time, find the ascending diminished 7th. Again, use the C-sharp major scale, counting up to the major 7th, but this time diminish by two half steps. An ascending diminished 7th from C-sharp is B-flat.
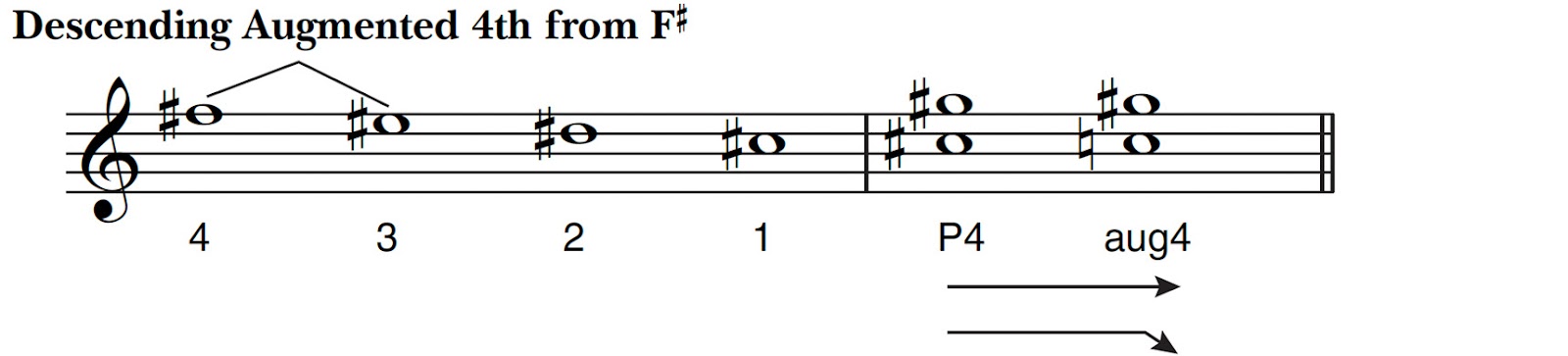
For descending intervals, we are given the higher note. We find the perfect or major descending interval first, counting down the major scale. Then we move the lower note to fit the exact quality we need.
If a minor or diminished interval is desired, we raise the lower note, moving it closer to the higher note, thus reducing the size of the interval.
If an augmented interval is desired, we drop the lower note farther away from the higher one, increasing the interval.
After constructing these intervals, we also need to make sure we can identify them. In this case, we can start from the lower note and avoid any backward counting.
Start with the major scale from the lower note. If the higher note is a member of the scale, the interval is perfect or major in quality.
If the higher note is not a member of the lower note's major scale, then count how many half steps away the note is from the scale member with the same letter name. If it is higher, the interval is augmented. If it is lower, the interval is minor or diminished.
Abbreviating Interval Names
A perfect or major interval is implied by not stating the quality. For example, a 2nd is always a major 2nd unless you say it is minor, augmented, or diminished. The same applies to 3rds, 6ths, and 7ths. A unison, 4th, 5th, or octave interval is always perfect unless it is called something else.
The word "sharp" and its symbol (picture below) is used to mean augmented. For instance, a sharp-5 is an augmented 5th.
The word "flat" and its symbol (pictured below) are used to mean minor when applied to a 2nd, 3rd, 6th, or 7th. When applied to a perfect interval (1, 4, 5, or 8), "flat" means diminished.
When a major interval is diminished, it can be written with two flats (symbol pictured below) instead of dim or d or the diminished symbol (pictured below).
Interval Mnemonics
You may find interval recognition easier when you can compare what you are singing to a familiar melody. Here are some traditional references to help remember interval sounds. It only works if you can really sing the little melodies, so you might need to practice that at first.
Notice that the first note of a melody is not always the tonic note! For instance, in "Happy Birthday," the major 2nd interval is from the 5th to the 6th in the major scale.
If these songs are unfamiliar to you, find and learn them as part of your music education. Also listen for the intervals in other songs you know.
Make interval recognition part of your daily practice. It’s the foundation for transcription of melodies and chord progressions.
Compound Intervals
Compound intervals are larger than an octave. They can be considered equivalent to their counterparts an octave lower, which are called simple intervals. The 9th interval, for instance, is a compound 2nd (a 2nd plus an octave) and is likewise major in quality.
From the newest releases to award-winning bestsellers and everything in between, Sheet Music Direct is your home for premium sheet music, all available to download and print instantly from any device.
Enjoy unlimited online sheet music, plus 50% off prints with PASS. Start your free trial now.

































Leave a Comment